Table of Contents
How to find critical value? Critical value is a concept in mathematics that helps us find the points at which a function becomes undefined. It’s also known as the point of inflection or the point of maximum deviation.
In most cases, critical values can be found by solving an equation or plotting the graph. However, there are some instances where you won’t be able to solve the equation or plot the graph, and you’ll have to use a different method.
What is critical value?
A critical value is the threshold at which a particular statistical test will declare that the hypothesis being tested is not supported. The critical value is often determined by the level of significance that is set for the test.
For example, if a researcher sets a level of significance at 0.05, then any value that is less than or equal to 5% of the total number of data points will be considered a statistically significant result.
What’s the importance of critical value?
A critical value is the cutoff point for a statistical test. In other words, it is the value below which the results of a test are considered to be statistically significant.
Critical values are important because they allow researchers to determine whether or not their results are due to chance. If the results of a test fall below the critical value, then it can be concluded that they are not due to chance and that the hypothesis being tested is actually true.
How to calculate critical value
Compute the alpha value
The alpha value (α) is the probability of making a Type I error. It is also known as the significance level.
To compute the alpha value, you need to know the Type I error rate (β), the power of the test (1-β), and the sample size (n).
Calculate the critical probability
The critical probability is a measure of how likely it is that a statistic computed from a sample will be greater than or equal to the critical value. The critical value is the cutoff point for the test statistic, and the critical probability is the likelihood that the test statistic will be greater than or equal to the critical value.
Use the critical t statistic for small sample sets
The critical t statistic is used to determine the significance of a difference or correlation in a small sample set. This statistic is determined by the degrees of freedom and the level of confidence.
The critical t statistic is used to determine if the difference or correlation found in the sample set is significant.
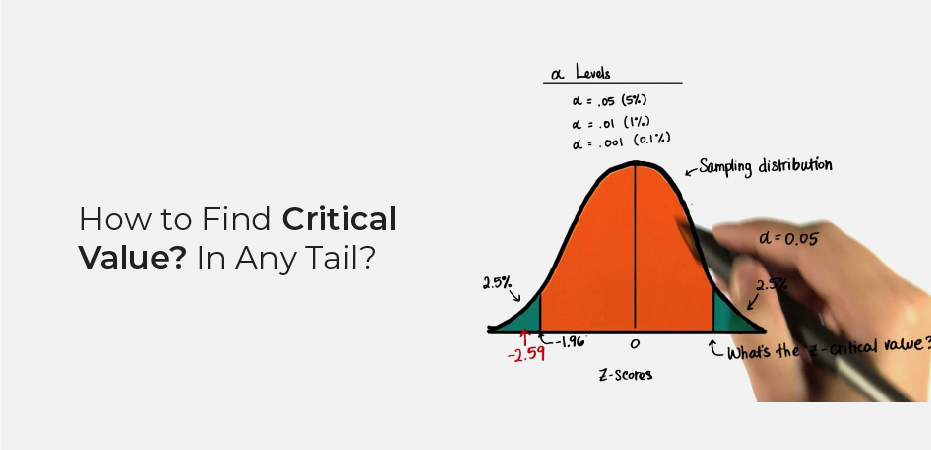
Express critical value as a Z-score for large data sets
The critical value is an important concept in statistics. It is the value that separates the significant results from the insignificant results. The critical value can be expressed as a z-score for large data sets. This makes it easy to determine whether or not a result is significant.
Types of critical value systems
Chi-squares
A critical value is the cutoff point for a Chi-squared statistic. The Chi-squared statistic is used to determine whether or not there is a significant difference between the observed values and the expected values.
If the Chi-squared statistic is greater than the critical value, then there is a significant difference between the observed values and the expected values.
T-scores
A critical value is the cutoff score used to determine whether or not a test result is significant. The most common type of critical value is the T-score, which is used to measure the difference between a test result and the average score for a group of people.
A T-score of +1.96 is used to determine whether or not a test result is statistically significant, meaning that it’s unlikely to have occurred by chance.
Z-scores
The critical value is the cutoff score on a test that determines whether or not a student has passed. The Z-score is the measure of how far a student’s score is from the mean score. A Z-score of 0 means that the student’s score is exactly the mean, while a Z-score of 1 means that the student’s score is one standard deviation above the mean. A Z-score of -1 means that the student’s score is one standard deviation below the mean.
Example
An important concept in statistics is the critical value. This is the value below which the probability of a Type I error (false positive) is exceeded. The critical value is determined by the significance level and the sample size.
For example, if you are using a significance level of 0.05 and you have a sample size of 100, then the critical value would be 1.96. This means that if your statistic falls below 1.96, there is only a 5% chance that it was due to chance and not to some real difference in the population.
FAQs
Q: How do you find the critical z value?
A: The critical z value is the cutoff point for a confidence interval. To find it, you need to know the margin of error and the sample size. You can use a calculator or an online tool to help you with this.
Once you have the critical z value, you can use it to determine whether or not your results are statistically significant.
Q: What is a critical value and where do you find it?
A: Critical values are important in statistics because they are used to determine whether or not a data set is unusual. This helps researchers to understand if their data is reliable and how it compares to other data sets. Critical values can be found in statistical tables, which are available online or in textbooks.
Q: What is the critical value of 95?
A: In statistics, the critical value of 95 is the threshold point at which the probability of a Type I error (false positive) is equal to 5%. Here (1-0.95)/2 = 0.025.
Q: What is the Z critical value for a 95 confidence interval?
A: In statistics, the Z critical value is the cutoff point for a 95% confidence interval. If the calculated Z value is greater than or equal to the Z critical value, then the confidence interval is considered statistically significant.
If the calculated Z value is less than the Z critical value, then the confidence interval is not considered statistically significant. Z=1.96